Surlethe wrote:Mathematically, we can deal in many, many dimensions; it's just as easy as defining a new axis into existence at right angles to the others. For example, let v = <v_1,v_2,v_3,...,v_n>. Then v = <v_1,v_2,v_3,...,v_n>, where each component is at right angles to each other one.
One can define a new orthogonal axis, true enough, but your statement is somewhat misleading--just because there is another coordinate does not mean that it is orthogonal to the rest, even assuming that there is some sort of inner product that lets one compute distances and angles between vectors.
Surlethe wrote:Now, the hard part is visualizing a vector like that.
Ironically, sometimes introducing more dimensions can actually help in visualizing a hyperdimensional object, or at least aid in understanding. As a simple example, suppose one has the equation a²+b²+(a-b)² = c²+d² over the reals. One can interpret it as a three-dimensional surface in four-dimensional (Euclidean) space, but it might not be immediately obvious as to what kind of geometry it has. But if there is a particular (a,b,c,d) that satisfies this equation, then both sides of it are equal to some particular positive number, say e². One has introduced another axis, creatively named e. Concentrating on what happens to the surface as one varies e, one in fact has two independent equations for any fixed e: a²+b²+(a-b)² = e², c²+d² = e². But the former is an ellipse in (a,b)-plane and the latter is a circle in (c,d)-plane. Effectively, the hypersurface has been "split" into two a pair of ordinary three-dimensional objects that are "connected" through e: (a,b,e)-space and (c,d,e)-space, both of which form double-cones (one elliptic, the other circular). Instead of trying to imagnie the complete object in four dimensions, one can concentrate on two separate surfaces in those two 3-spaces and consciously think of them as connected. Another possible method is to take a plane, say (c,d), and think of each point as 'leading to' a unique plane (a_c,b_d) in which there is an ellipse. This is easier to generalize to higher number of dimensions, but less visual.



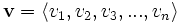 .
.