Congruent Triangles?

[Surlethe]

Damn you, Elheru Aran, for bringing this to my attention!



Okay, this is driving me crazy. The area of the bottom triangle is apparently the area of the top minus one. However, both areas are incorrect: the value obtained by counting sides and applying the formula for a triangle's area is a fraction, but the area of each constituent part is an integer, so both triangles are off by a half unit. Thus, I conclude it must be an optical illusion, but I have no idea how it works or how it can apparently lose an entire unit through a rigid rearrangement.

[Kuroneko]

The angles are subtly mismatched. Calculate the bottom-left angles of the red and dark green pieces. If you wish, also compare them with with the large triangles. You'll see the difference.

[Gil Hamilton]

The different configuration allows the hole. The total length of the overall triangle is 13 units and 5 units high. When you switch the bottom red triangles positions, the space underneath the red triangle is a rectangle 8 units wide and 2 units high. You can make a rectangle of said dimension out the remaining "L" pieces, but interlocking the narrow points rather than the entire thing. This makes an 8 unit length on the top, but the 2 length of the yellow peices "L" and the 5 length of the long part of the green "L" adds up to seven, so there is a space.

It makes alot of sense to me, though it's a neat kink of configuration. Do it with cutout pieces of paper. Because I'm a media arts major rather than a mathematician, I broke out the straight edge and exacto knife and recreated that way.

At any rate, the missing gap comes from the different way of interlocking the "L" pieces

[Gil Hamilton]

The angles are subtly mismatched. Calculate the bottom-left angles of the red and dark green pieces. If you wish, also compare them with with the large triangles. You'll see the difference.

I don't think that the angles are subtly different. I just got done cutting the drawing the pieces and with the exact same pieces, arranged them in both configurations.

[Cyborg Stan]

Of the long sides, the smaller one has a slope of 2/5, the other a slope of 3/8. Of course the angles would be different. Had the dark-green piece been stretched out to a similar triangle of length 13, it would've been 5.2 units high. For the red piece, 4.875.

Put it another way, you just have a quadrilateral trying to be sneaky.

[Kuroneko]

It makes alot of sense to me, though it's a neat kink of configuration. Do it with cutout pieces of paper. Because I'm a media arts major rather than a mathematician, I broke out the straight edge and exacto knife and recreated that way.

And that's also why you are wrong. The difference in area comes from the fact that in making your arrangement, you are either ignoring the gaps between the pieces or overlaps (depending on the arrangement you started with). If you started with a five-by-thirteen triangle, then the resulting pieces are not their specified dimensions. Had you started with the pieces first (cut them out before making the large triangles), you would not be able to make a triangle with them without some gaps or overlaps.

I don't think that the angles are subtly different. I just got done cutting the drawing the pieces and with the exact same pieces, arranged them in both configurations.

Bottom-left angles: atan(2/5) = 21°48'05" (red triangle), atan(3/8) = 20°33'22" (dark green triangle), none of them matching atan(5/13) = 21°02'15" (large triangle). This is nothing but a clever play on pieces with angles that are close together but not actually matching.

[brianeyci]

Yay easy math!

arctan(3/8 ) != arctan (2/5)

QED.

Yes I "cheated" and took Kuroneko's hint, but you'd be stupid not to take his hints.

Brian

[Beowulf]

Or you could take a look and note that at a horizontal position of 5 units from the left tip of the red triangle, it doesn't quite reach 2 units in height. Therefore the red triangle must have a smaller angle.

[Gil Hamilton]

And that's also why you are wrong. The difference in area comes from the fact that in making your arrangement, you are either ignoring the gaps between the pieces or overlaps (depending on the arrangement you started with). If you started with a five-by-thirteen triangle, then the resulting pieces are not their specified dimensions. Had you started with the pieces first (cut them out before making the large triangles), you would not be able to make a triangle with them without some gaps or overlaps.

Bottom-left angles: atan(2/5) = 21°48'05" (red triangle), atan(3/8) = 20°33'22" (dark green triangle), none of them matching atan(5/13) = 21°02'15" (large triangle). This is nothing but a clever play on pieces with angles that are close together but not actually matching.

I'm not contesting your math, but I assure you I cut the pieces to the exact unit diminsions shown in the illustration. I measured and drew each shape in the triangle exactly and cut it them out with an exacto knife. I only made one triangles worth of pieces, but I was able to make both arrangements with the pieces I had made, without overlapping. Both triangles measured 13 cm in length and 5 cm in height.

In fact, when I drew the triangle, I drew a 13 cm by 5 cm triangle and then cut the pieces from it.

If I had my digital camera in my apartment rather than at home I'd show you. If you want, cut the pieces out with the diminsions shown in the picture and make the arrangements yourself. You'll see that it can be done.

[brianeyci]

The difference between the angles is less than half a degree.

Unless you're superhuman with that exacto knife they'll be a mistake and overlap. I think if you made really large triangles maybe five inches to one of those units you'd easily see the overlap.

Look at it Beowulf's way...



Brian

[Kuroneko]

I'm not contesting your math, but I assure you I cut the pieces to the exact unit diminsions shown in the illustration.

Nonsense. This trick only works because of the inexactness of measurement.

If I had my digital camera in my apartment rather than at home I'd show you.

All you would show me is that either your triangle pieces do not detect an angle difference of 46' (about 3/4 of a degree) or fudged their dimensions by a bit less than a millimeter. That's quite easy to do when you're cutting by hand.

[mr friendly guy]

OK, so let me see if I got this right from other people's explanations.

The first "triangle" isn't really a triangle. Its a quadrilateral. You can find this out by taking the tangents of the red and green smaller triangles. So where the red triangle meets the green triangle is another side, but its so small we don't perceive it as another side (in fact I tried saving it as a jpeg and magnifying the image as far as it can go, but it still looks the same).

By the same token, the second "triangle" is also a quadrilateral. The extra side of course is where the green and red triangles meet. However as the triangles this time meet 6 squares from the edge instead of 9 squares, its a different quadrilateral. So this extra square must be appear presumably because this second quadrilateral is larger.

[Dooey Jo]

The optical "illusion" is caused by the grid. If you remove it, it becomes very clear that the parts do not form a triangle. With that in mind, you can easily calculate the areas of the two shapes (ignoring their constituent cut-out parts, of course).

The area of the first shape is
A = 5 * (3+5) / 2 + (3*8) / 2 = 32

The second
A = 8 * (2+5) / 2 + (5*2) / 2 = 33

Therefore, you will not be able to form the second shape by cutting the first one up into pieces and re-arranging them.

[Dooey Jo]

And that "cool" smiley is supposed to be 8). Sorry about that (should've disabled smilies).

[Molyneux]

OK, so let me see if I got this right from other people's explanations.

The first "triangle" isn't really a triangle. Its a quadrilateral. You can find this out by taking the tangents of the red and green smaller triangles. So where the red triangle meets the green triangle is another side, but its so small we don't perceive it as another side (in fact I tried saving it as a jpeg and magnifying the image as far as it can go, but it still looks the same).

By the same token, the second "triangle" is also a quadrilateral. The extra side of course is where the green and red triangles meet. However as the triangles this time meet 6 squares from the edge instead of 9 squares, its a different quadrilateral. So this extra square must be appear presumably because this second quadrilateral is larger.

Actually, each quadrilateral has exactly the same area (because they are composed of the same components); it's just like chopping a corner off of a square and gluing it onto another side. The area is the same, but its distribution is different.

[Gil Hamilton]

All you would show me is that either your triangle pieces do not detect an angle difference of 46' (about 3/4 of a degree) or fudged their dimensions by a bit less than a millimeter. That's quite easy to do when you're cutting by hand.

I'm not sure what we are arguing about, but I think I see the problem. I said that I didn't contest your math at all. I mean, I've taken trigonometry classes before, you know. Twice, actually, and not because I failed it the first time. I absolutely agree that the initial arrangement isn't a perfect triangle, even though it is triangularly arranged (I'm not sure how one can argue that it's not a triangular arrangement, it's alot more triangular than most things that I see called a "triangular arrangement").

What I'm saying is that if you cut out the pieces exactly to the diminsions shown in the illustration, which is what I did within human tolerances, you can make the above arrangement both ways with the pieces given, hence it is no mystery where the hole comes from, because the hole is made by how you interlock the two "L" pieces. Doesn't that answer the question? The puzzle asks where the hole comes from by rearranging the exact same pieces in what is basically the same perimeter as far as a person can see with the objects that size and the thick black lines drawn around the pieces (I'm sure your finger is itching to say not exactly the same, but this too is something I'm aware of). The way the two pieces interlock is the answer.

[brianeyci]

What I'm saying is that if you cut out the pieces exactly to the diminsions shown in the illustration, which is what I did within human tolerances,

There's either cut exact or not cut exact and if you cut within human tolerances you're not cutting exact most likely.

If you zoomed in on the vertex between the red and green triangles there wouldn't be a perfect line. So it's not a triangle. If something appears to be a triangle and is really a quadlateral it's not a triangle.

Also the context... since they use a grid it seems they want some kind of mathematical or scientific explanation. So the answer is the accuracy of the measurements are wrong. Especially since they imply with a grid that the measurements are right.

Brian

[Gil Hamilton]

There's either cut exact or not cut exact and if you cut within human tolerances you're not cutting exact most likely.

Cut exact means that when I measured the pieces with my straight edge, they were on the lines indicating that they were the length I wanted them to be. Nothing can cut exactly, not even a plotter with a razor on it.

If you zoomed in on the vertex between the red and green triangles there wouldn't be a perfect line. So it's not a triangle. If something appears to be a triangle and is really a quadlateral it's not a triangle.

Also the context... since they use a grid it seems they want some kind of mathematical or scientific explanation. So the answer is the accuracy of the measurements are wrong. Especially since they imply with a grid that the measurements are right.

You know the difference between "a triangle" and "triangular", don't you? Things that are triangular are very rarely perfect triangles, in nature, art, or math. For instance, I've used an oscilloscope to measure waveforms that were definitely triangular, in fact if I labelled them anything else I would have misidentified them, but were by no means composed in any way shape or form of real triangles. I suppose I shouldn't have used triangle at all in my previous posts, but the thing is definitely a triangular arrangement of individual units.

Besides, the puzzle doesn't give any context. The grid is necessary to establish units, but it says nothing about mathematics. The puzzle is only effective because it is not mathematically sound, as you all have proved very adequately. The way the question is presented on the sheet is straight forward enough, it asks where does the hole come from. That is obvious, it comes from a different arrangement of the "L" shapes. With the units shown, it happens to fit into very close to the same perimeter, therefore making it "neat".

[brianeyci]

Cut exact means that when I measured the pieces with my straight edge, they were on the lines indicating that they were the length I wanted them to be. Nothing can cut exactly, not even a plotter with a razor on it.

I believe exact's used in science it means with such a high degree of accuracy and precision that you cannot with your instruments find any experimental error. I'm sure you already know this. Since I can tell with my eye that the thing isn't exact, it's not (the puzzle, not your cutouts).

You know the difference between "a triangle" and "triangular", don't you? Things that are triangular are very rarely perfect triangles, in nature, art, or math.

I guess I approach always from a math angle.

With the units shown, it happens to fit into very close to the same perimeter, therefore making it "neat".

Why do you quote neat? It's because you know that it's not really neat, that it's not really exact. I still think the most straightforward answer is that the pieces are not exact, or not accurate enough, given the context of the grid implying exactness. You don't need math to tell this--you can just eyeball it.

Saying that rearranging the pieces is the answer is missing the whole point of the "trick", that's why I think it's wrong.

Brian

[Zadius]

Simply put, the green triangle has a slightly steeper slope than the red one.

[Gil Hamilton]

I believe exact's used in science it means with such a high degree of accuracy and precision that you cannot with your instruments find any experimental error. I'm sure you already know this. Since I can tell with my eye that the thing isn't exact, it's not (the puzzle, not your cutouts).

This reminds me of an Engineering joke my Calculus teacher told me. Two men die in a car crash, one is a Mathematician, one is an Engineer. St. Peter says they may see their dead wives, but they may only walk to them by crossing half the distance between them and the wife each time. The Mathematician dispairs and cries to St. Peter "But I will never reach her! How can you be so cruel?". The Engineer walks the distances and easily reachs his wife, then turns to St. Peter and the Mathematician and shrugs. "Close enough" he says.

13cm with a straightedge and pencil means that you draw a line that anyone with a straightedge can measure to be 13cm.

I guess I approach always from a math angle.

Heh, that's a good pun.

Why do you quote neat? It's because you know that it's not really neat, that it's not really exact. I still think the most straightforward answer is that the pieces are not exact, or not accurate enough, given the context of the grid implying exactness. You don't need math to tell this--you can just eyeball it.

Saying that rearranging the pieces is the answer is missing the whole point of the "trick", that's why I think it's wrong.

Brian

"Neat" as in "interesting", not as "neat" as in "clean".

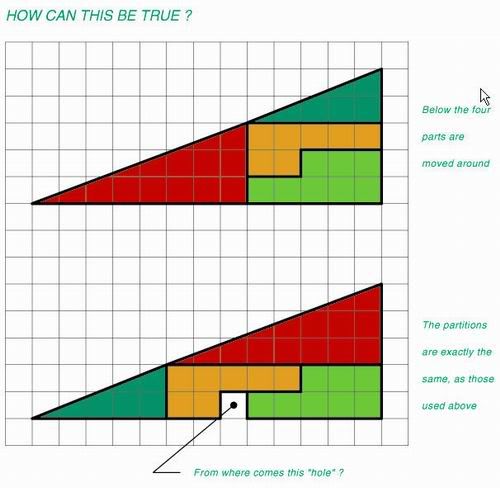
But remember, the above or the cutout I made aren't science or math. In fact, the puzzle seems designed specifically to those who overanalyze the situation. Surlethe originally was confounded because the thing didn't make any mathematical sense. You can approach it mathematically, of course, but it is very simple to say that when you move the red block to the top corner of the triangular arrangement, in order to maintain the general shape of the arrangement you are left with a rectangle under the the red triangle that is 8 by 2 units. You can make a rectangular arrangement of shapes with the two "L" pieces matching those diminsions, but you are left with the hole. Hence, the hole comes from the different configuration of the "L" pieces in the total triangular arrangement.

If anything about the above is untrue, feel free to point it out. However, the key to the puzzle is to not walk into the trap of overthinking it. It asked you a simple question, and has a answer so simple that most people just overlook it.

[Braedley]

Jeez Surly, you should be ashamed of yourself for not figuring it out I took me all of five minutes the first time I say it.

[mr friendly guy]

OK, so let me see if I got this right from other people's explanations.

The first "triangle" isn't really a triangle. Its a quadrilateral. You can find this out by taking the tangents of the red and green smaller triangles. So where the red triangle meets the green triangle is another side, but its so small we don't perceive it as another side (in fact I tried saving it as a jpeg and magnifying the image as far as it can go, but it still looks the same).

By the same token, the second "triangle" is also a quadrilateral. The extra side of course is where the green and red triangles meet. However as the triangles this time meet 6 squares from the edge instead of 9 squares, its a different quadrilateral. So this extra square must be appear presumably because this second quadrilateral is larger.

Actually, each quadrilateral has exactly the same area (because they are composed of the same components); it's just like chopping a corner off of a square and gluing it onto another side. The area is the same, but its distribution is different.

Ah, no. They can't be composed of the same components as the second quadrilateral has an extra white square.

[Jaepheth]

Top U Middle = Bottom




the area of the quadrillateral created by the differences of the "hypotenuses" of the "triangles" is given by

|<8,3>x<5,2>|=

| i j k |
| 8 3 0 | = i(0)-j(0)+k(16-15) = |k| = 1
| 5 2 0 |



the difference in area at the "hypotenuse" = exactly 1 unit.

[Star-Blighter]

This has been a most (unintentionally) amusing thread. First looking at the puzzel the answer seemed pretty intuitive, and then I started reading the following posts...

Much confusion to be had by me.

SD.net. The only place where even the most trivial of geometry puzzels are held to rediculously exacting scientific standards. Great work on the overlays though.